
1. Show that in a right angled triangle, the hypotenuse is the longest side.
solution :

Given,
A right triangle
Find,
hypotenuse is the longest side
In the Fig.,
we know that,
∠A + ∠B + ∠C = 180°
Now,
∠B + ∠C = 90°
Then,
∠A = 90°
∴ ∠A = ∠B + ∠C
∴ ∠A > ∠B
Similerly
∴ ∠A > ∠C
∴ BC > AC
Similerly
∴ BC > AB
∴ BC is the longest side.
So, hypotenuse is the longest side.
2. In Fig. 7.48, sides AB and AC of ` \▵\ `ABC are extended to points P and Q respectively. Also, ∠PBC ` \<\ ` ∠QCB. Show that AC ` \>\ ` AB.
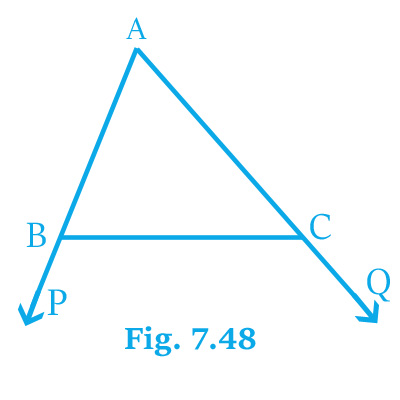
solution :
Given,
Side AB and AC of ` \▵\ `ABC are extended to points P and Q respectively. also, ∠PBC < ∠QCB.
Find,
AC > AB
In the Fig.,
∠ABC + ∠PBC = 180°[ Linear pairs of angles ]
∠ABC + = 180° − ∠PBC ` \-----(I)\ `
Also,
∠ACB ∠QCB = 180°[ Linear pairs of angles ]
∠ACB = 180° − ∠QCB ` \-----(II)\ `
From (I) and (II)
∠ABC > ∠ACB
Hence,
AC > AB as sides opposite to the larger angle is always larger.
3. In Fig. 7.49, ∠B ` \<\ ` ∠A and ∠C ` \<\ ` ∠D. Show that AD ` \<\ ` BC.

solution :
Given,
∠B < ∠A and ∠C < ∠D.
Find,
AD < BC
In the Fig.,
∠B < ∠A[ Given ]
AO < BO ` \----- (I)\ `
∠C < ∠D[ Given ]
OD < OC ` \----- (II)\ `
From (I) and (II)
AO + OD < BO + OC
AD ∠ BC
4. AB and CD are respectively the smallest and longest sides of a quadrilateral ABCD(see Fig. 7.50). Show that ∠A ` \>\ ` ∠C and ∠B ` \>\ ` ∠D.
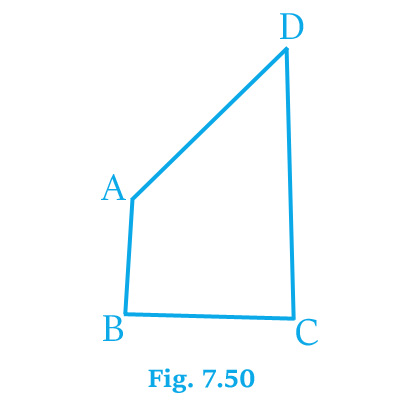
solution :
Given,
AB and CD are respectively the smallest and longest side of a quadrilateral ABCD.
Find,
∠A > ∠C and ∠B > ∠D.
Contruction,
Join AC and DB.

In ` \▵\ `ABC,
AB < BC[ Given AB is smallest line ]
∠ACB < ∠BAC ` \-----(I)\ `[ Angle opposite to longer side is greater ]
In ` \▵\ `ACD,
AD < DC[ Given CD is longest line ]
∠ACD < ∠DAC ` \-----(II)\ `[ Angle opposite to longer side is greater ]
Adding (I) and (II), we get
∠ACB + ∠ACD < ∠BAC + ∠DAC
⇒ ∠BCD < ∠BAD
SO, ∠A > ∠C
In ` \▵\ `ABD,
AB < AD[ Given AB is smallest line ]
∠ADB < ∠ABD ` \-----(III)\ `[ Angle opposite to longer side is greater ]
In ` \▵\ `BCD,
BD < DC[ Given CD is longest line ]
∠BDC < ∠DBC ` \-----(IV)\ `[ Angle opposite to longer side is greater ]
Adding (III) and (IV), we get
∠ADB + ∠BDC < ∠ABD + ∠DBC
⇒ ∠ADC < ∠ABC
SO, ∠B > ∠D
5. In Fig. 751, PR ` \>\ ` PQ and Ps bisects ∠QPR. Prove that ∠PSR ` \>\ ` ∠PSQ.

solution :
Given,
PR > PQ and PS bisect ∠QPR
Find,
∠PSR > ∠PSQ
In ` \▵\ `PQR,
PR > PQ[ Given ]
∠PQR > ∠PRQ ` \-----(I)\ `[ Angle opposit to longer side to greater ]
PS is the bisector of ∠QPR
So, ∠QPS = ∠RPS ` \-----(II)\ `
In ` \▵\ `PQS,
∠PSR = ∠PQS + ∠QPS ` \-----(III)\ `[ The exterior angle of a triangle equals to the sum of opposite interior angles ]
In ` \▵\ `PSR,
∠PSQ = ∠PRS + ∠RPS ` \-----(IV)\ `[ The exterior angle of a triangle equals to the sum of opposite interior angles ]
Addind (I) and (II), we get
∠PQR + ∠QPS > ∠PRQ + ∠RPS
From (III) and (IV),
∠PSR > ∠PSQ
6. Show that of all line segments drawn from a given point not on it , the perpendicular line segment is the shortest.
solution :
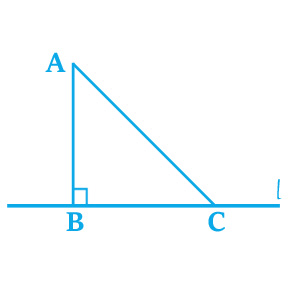
Given,
l is a line and A is a point not lying on l. AB ⊥ l. C is any point on l other than B
Find,
AB < AC
In ` \▵\ `ABC,
∠B = 90°
⇒ ∠C is an acute angle.[ Angle sum property of a triangle ]
⇒ ∠B > ∠C
⇒ AC > AB[ Side opposite to greater angle is greater ]
So, AB < AC.




0 Comments